I do soft matter physics; see how P.-G. de Gennes defined soft matter in his Nobel lecture in 1991.

Why soft matter?

One of the most remarkable characteristics of physical systems that make up the so-called soft condensed matter (liquid crystals, polymers, gels, foams, colloids, membranes, granular media...) is that in spite of their diversity, they can all be described within a more or less common framework consisting of a few theoretical concepts. On top of that, soft materials are earthly: they occur at (or close to) the room temperature and are often encountered in everyday life, and some of them actually constitute living organisms. Many soft matter systems can be studied with tabletop experimental equipment, and this provides access to interesting physics at a reasonable cost. In my view, these are the main two attributes that make the physics of soft condensed matter so attractive.

In 2005, RSC launched
a specialized journal to provide
"a forum for the communication
of generic science underpinning
the properties and applications
of soft matter"

Soft matter is also important from a purely theoretical perspective: Given that in many systems, interactions between constituents can be tuned to a large extent and the dimensionality can be often easily reduced from 3 to 2 and 1 spatial dimension, it is an excellent testbed for statistical-mechanical and field-theoretical models.

In the past decade, soft matter systems became increasingly more attractive for a broad range of applications. This is partly due to ever more sophisticated control of their self-assembly, which can produce sizable amounts of engineered materials with desired mechanical, thermodynamical, electrical, and optical properties. The other reason that makes soft matter more and more appealing is the advent of nanotechnology as the umbrella name for all the different tools for experimental analysis, manipulation, and assembly of nanoscale objects, many of which belong to a class of materials listed above

Liquid crystals

Liquid crystals are no exception to the above, and a considerable part of my work was and still is focused on liquid crystals; more specifically, on liquid crystals in confined geometries. The theoretical approach that I use is based on a mesoscopic description the liquid-crystalline order and a corresponding phenomenological thermodynamic or elastic theory. Topics I have studied were mainly limited to nematics, the simplest type of liquid crystals.

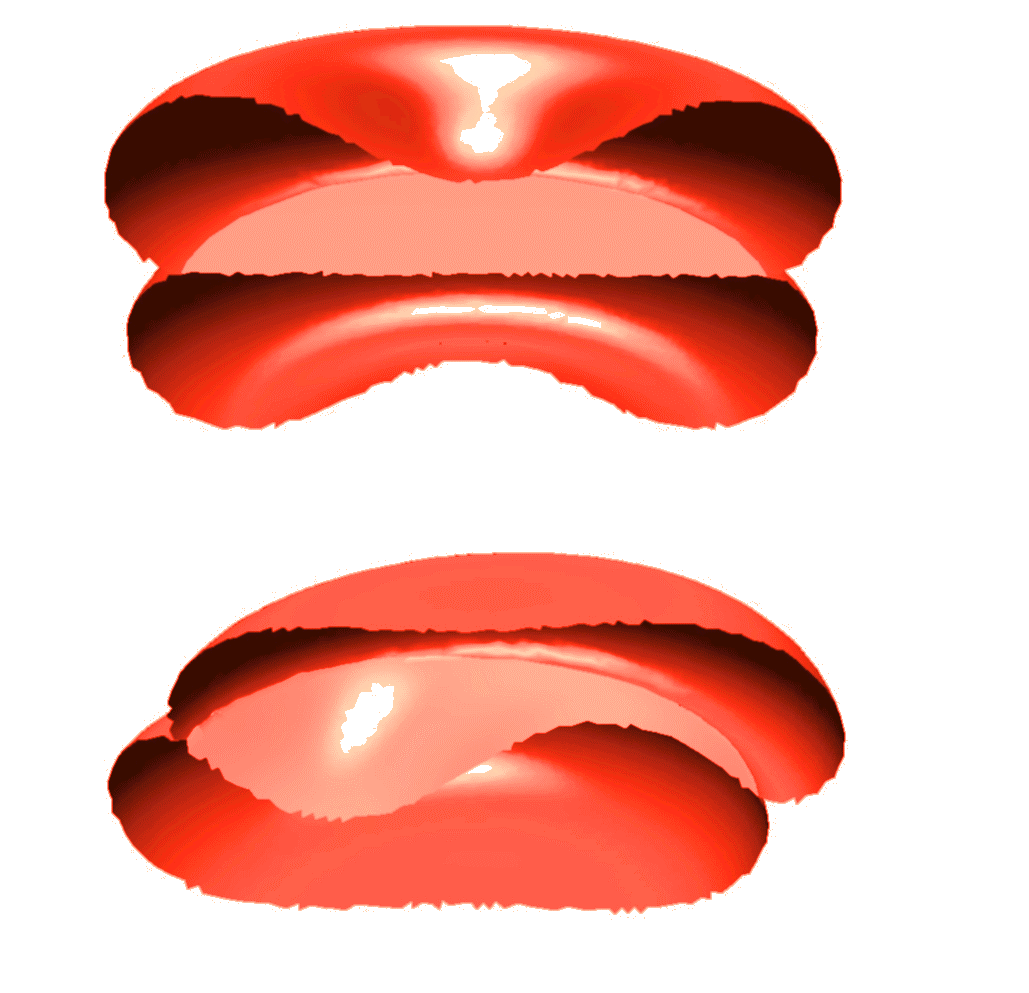
Much of my work in this field has to do with fluctuations of the various types of liquid-crystalline order, especially at structural and phase transitions where the analysis of soft modes can be used to examine the stability of the system in question. Also interesting are fluctuations in the various wetting geometries where the soft mode is spatially localized and corresponds to the fluctuations of the interface between the wetting layer and the bulk phase.

Another related effect is the fluctuation-induced (aka Casimir) force; a very intriguing phenomenon, partly due to its universality. But in real liquid-crystalline systems, the universal behavior of the force may not be apparent due to finite anchoring strength, and it is worth studying in its own right. Experimentally, this force is probably most obvious in very thin nematic films which are frustrated by the competing aligning effects of the substrate and the free surface. As a result, the structural interaction can destabilize the film and lead to very interesting dewetting morphologies, and we have shown that the interaction in question could be due to fluctuations. The story does not seem to be quite closed though.

Colloids

Physics of colloids can be enormously complex, but also very simple. The most stripped-down way of looking at colloidal particles is to think of them as of a system of noninteracting hard spheres. Then they should form a liquid phase at low densities and an FCC crystal at large densities. Now the question is what would be the symmetry of a colloidal crystal formed by particles that are just a little different from hard spheres? This is not just an academic question: Work along these lines may be useful for the fabrication of 3D photonic crystals by colloidal self-assembly. The crystal lattice best suited for photonic applications is the notoriously open diamond lattice...

In a heuristic way, this problem can be modeled by decomposing the free energy of the crystal into a bulk part that corresponds to the hard-core interaction and a "interfacial" part that accounts for the soft tail of the potential. The former is minimized by the FCC lattice, the latter by the so-called A15 lattice; BCC is somewhere in between. Using this approach, we were able to interpret the stability of several non-close-packed lattices in a number of systems such as dendrimers, diblock copolymers, aurothiols, etc.

The problem of pinning down the necessary conditions an interparticle potential should satisfy to render the close-packed lattice unstable is still open. Perhaps this issue should first be addressed in 2 dimensions; the simulation studies provide plenty of data and ever more complex phases even in very simple systems...

J. Phys. Chem. B cover
with artwork announcing
our feature article in this issue

A related topic that we have modeled recently is colloidal aggregation in systems with repulsive pair potentials. For a certain type of potentials, colloids clumb into clusters such as micelles and lamellae, which then form an appropriate crystal lattice. (A very interesting effect; still largely unexplored.)

Phospholipid vesicles

The simplest model of the cell membrane is a phospholipid vesicle that divides the interior from the exterior. Many (though by far not all) morphological features of cells do in fact depend of the mechanics of the bilayer; the correspondence is most direct in red blood cells which have no internal structure.

The theoretical phase diagram of vesicles with all of the axisymmetric shapes such as diskocytes, stomatocytes, cigars, pears, budded shapes etc. has been known in some detail. But the part of the phase space separating the invaginated and the evaginated axisymmetric shapes has been explored only partly. Using a fully numerical procedure, we have analyzed some of the nonaxisymmetric shapes of vesicles, and a consistent structural hierarchy of combined shapes with disk-like body and one or more prolate arms - rackets, boomerangs, and starfish - was found.

Racket, boomerang, and starfish