Matej Pavsic
Home Page
Clifford Space as
a Possible Clue to the Unification of Fundamental Interactions
Geometric calculus based on
Clifford algebra is a very useful mathematical tool
for description of physics. In addition, it enables formulation of a
new theory
in which the 4-dimensional spacetime is replaced by a more
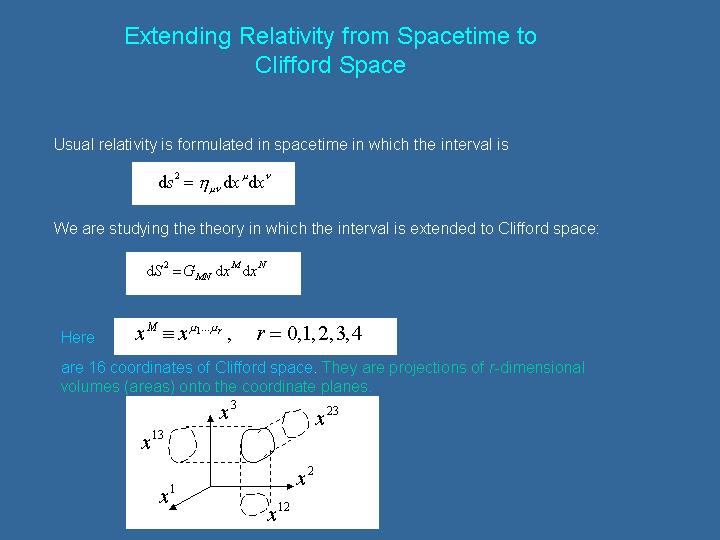
general, 16-dimensional space,
called Clifford space (C-space). This is a space whose elements are not
only points, but also lines,
areas, 3-volumes and 4-volumes. Such generalization brings
many new theoretical possibilities that
are being explored.
If we release the
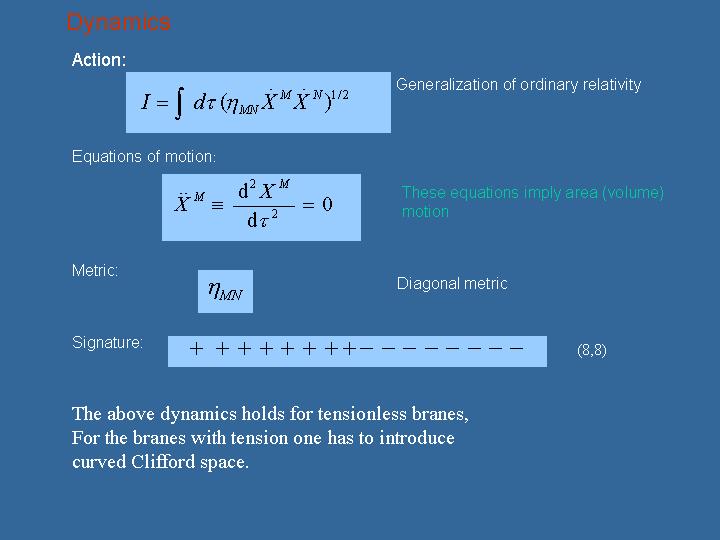
constraint of flat C-space, then we encounter a fascinating
possibility, namely
that curved C-space provides a realization of Kaluza-Klein theory
[ http://arxiv.org/abs/gr-qc/0507053 ],
and since all of its 16 dimensions are by assumption physically
observable, there is no
need for compactification of the ``extra'' dimensions.
The concept of C-space is
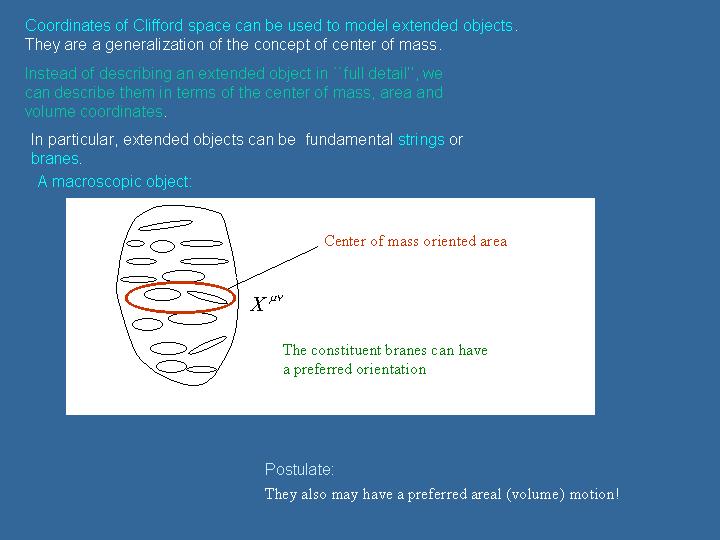
related to the concept of the configuration space associated
with the extended objects, the so called branes. [See
A Summary of the
Concept of the Configuration Space Associated with Extended Objects
(Branes) ].
A Brief Summary of the Concept of Clifford Space
A Summary of the
Concept of the Configuration Space Associated with Extended Objects
(Branes)
More reading
See
also other papers to be found on my Home Page